Home > Topdrawer > Mental computation > Good teaching > Addition and subtraction > Making choices
Making choices
Strategic choice involves deciding on the most efficient process to use and how the numbers will be manipulated. Successful mental calculation eases memory load by carrying out the operation in the smallest number of steps.
There are three types of problems for combining sets (addition) and partitioning sets (subtraction).
The most commonly encountered problem type is result unknown problems.
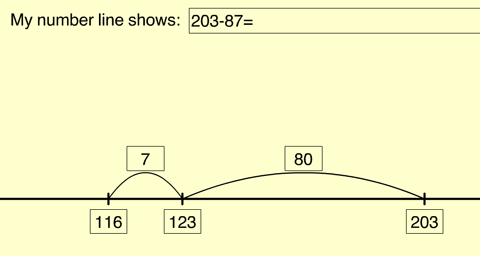
For example, 125 + 68 = ? and 203 – 87 = ? are result unknown problems. So the answer is the end result of the joining or separating.
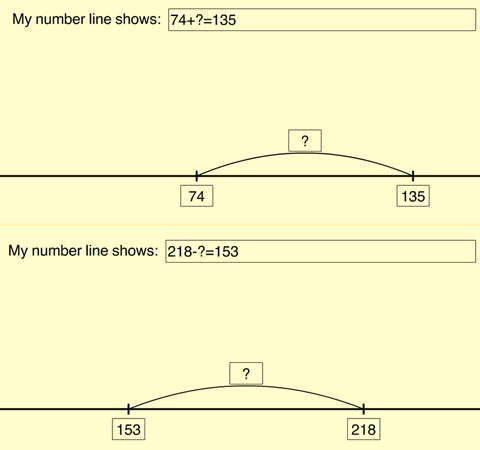
Change unknown problems require a student to find out what was added (joined) or subtracted (separated).
For example, 74 + ? = 135 and 218 – ? = 153 are of this type. These problems tend to be more difficult than result unknown problems given similar numbers.
Start unknown problems are the most difficult as they require the student to establish the starting state before a set was joined or separated.
For example, ? + 46 = 82 and ? – 56 = 37 are of this type. Solving these problems involves undoing an operation therefore seeing addition and subtraction as inverse operations.
You can read more in the articles Strategies for Going Mental and Mental Methods Moving Along on the AAMT website.