Home > Student activities > National Maths Day > National Maths Day 2014
National Mathematics Day 2014
Twist your brain on Friday 22 August with these paradoxes!
Some are counter-intuitive, some are tricky and others are just baffling!
Consider this:
Tomorrow never comes.
In the paradoxes below, click on More... to find the 'solution' or explanation. And if you have a favourite paradox, send it to us.
The birthday paradox
How many people do you need to have in a room for there to be a 50% chance of two people with a birthday on the same day (but not necessarily the same year)? How many to have a 99% probability of a match?
There only needs to be 23 people in a room for there to be a 50% chance of a shared birthday and only 57 people for there to be a 99% chance! Surprised?
Test it out by doing birthday surveys in your school. How many people before you get a match?
‘Proof’ that 2 = 1
let a = b, then a2 = ab
a2 - b2 = ab-b2
(a-b)(a+b) = b(a-b)
a+b = b, substituting gives b+b = b
2b = b therefore 2 = 1
Where is the error made?
Hilbert’s paradox of the Grand Hotel
The Grand Hotel has an infinite number of rooms, all of which are occupied. Can the hotel accommodate any more guests?
Does 0.999… = 1?
Sometimes this seems counter-intuitive. Try using fractions or algebra to show that this is true.
Zeno’s paradox
The finish line is 2 metres away. The runner must first reach half the distance to the finish line (1m) but when there must cover half of the remaining distance (1/2 m). Having done that the runner must cover half of the new remainder (1/4m), then 1/8m, then 1/16m etc.
Will the runner ever reach the finish line?
The potato paradox
You have 100kg of Martian potatoes, which are 99 percent water by weight. You let them dehydrate until they’re 98 percent water. How much do they weigh now?
A twist on Gardner's two children problem
Mr Jones has two children. The older child is a girl. What is the probability that both children are girls?
Mr Smith has two children. At least one of them is a boy. What is the probability that both children are boys?
The twist:
Mr Ng has two children. One is a boy born on a Tuesday. What is the probability that both children are boys?
The Monty Hall problem
You are a contestant on a game show. At the show's conclusion you are presented with three doors, each of which conceals a prize. Behind one of the doors is a car. Behind each of the other two doors is a goat.
After you have selected one of the doors, the host will open one of the two remaining doors to reveal a goat. At this point you will have the option of opening the door you originally selected and taking the prize behind it, or switching to the remaining unopened door and going home with the prize it conceals.
Is it in your best interest to switch? Will it improve your odds?
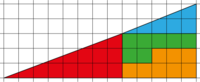
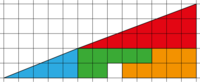
The missing square
Cut this triangle into pieces and reassemble it.
Why is there a missing square?
The two-envelope paradox
There are two envelopes, both containing money. One envelope has exactly twice the amount of money as the other. You can keep the money inside the envelope that you choose.
You pick one of the envelopes and open it. Inside is $20. Should you switch envelopes?