Home > Topdrawer > Patterns > Misunderstandings > Rectangular grids
Rectangular grids
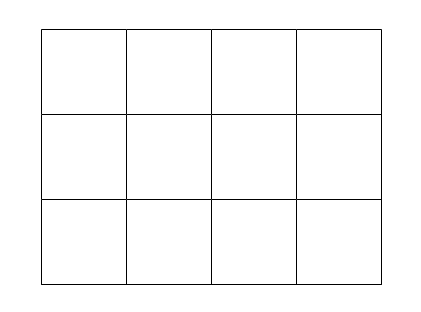
This rectangular grid is filled with 12 identical squares, with no spaces between them.
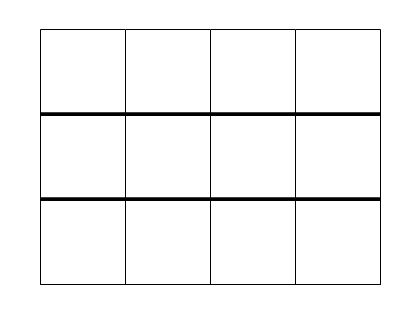
That same rectangular grid can be seen as three rows of four squares.
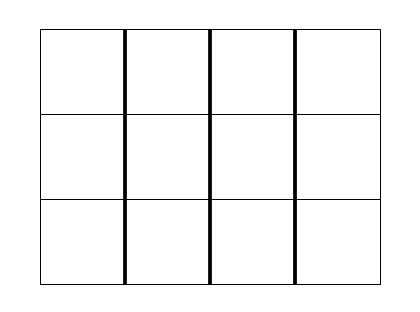
It can also be seen as four columns of three squares.
A rectangular grid is therefore a repeating pattern in two ways.
The rectangular grid can be a most valuable aid in early mathematics learning.
- It incorporates many spatial concepts (square, rectangle, congruence, collinearity, parallels and perpendiculars).
- It can provide an introduction to multiplication.
- Counting the number of squares leads to skip counting (4, 8, 12 and 3, 6, 9, 12 in the above grid).
- Counting the number of squares also leads to multiplication (4 \(\times\) 3 = 12 and 3 \(\times\) 4 = 12).
- This counting eventually leads to the formula for the area of a rectangle.
- It can be used to illuminate many multiplication, division and fraction situations.
The structure of the rectangular grid will not be immediately obvious to many students so it is important to teach it explicitly.
Structure of rectangular grids
Textbooks often use rectangular grids to illustrate multiplication, area and fractions. But research shows that young students may not understand the structure of rectangular grids.
Drawing rectangular grids
There are many practical and enjoyable drawing activities through which students can learn the structure of the rectangular grid.
Rectangular arrays
By studying rectangular arrays, students can also learn the structure of the rectangular grid pattern.